| comments | difficulty | edit_url | tags | |||
|---|---|---|---|---|---|---|
true |
Medium |
|
The boundary of a binary tree is the concatenation of the root, the left boundary, the leaves ordered from left-to-right, and the reverse order of the right boundary.
The left boundary is the set of nodes defined by the following:
- The root node's left child is in the left boundary. If the root does not have a left child, then the left boundary is empty.
- If a node in the left boundary and has a left child, then the left child is in the left boundary.
- If a node is in the left boundary, has no left child, but has a right child, then the right child is in the left boundary.
- The leftmost leaf is not in the left boundary.
The right boundary is similar to the left boundary, except it is the right side of the root's right subtree. Again, the leaf is not part of the right boundary, and the right boundary is empty if the root does not have a right child.
The leaves are nodes that do not have any children. For this problem, the root is not a leaf.
Given the root of a binary tree, return the values of its boundary.
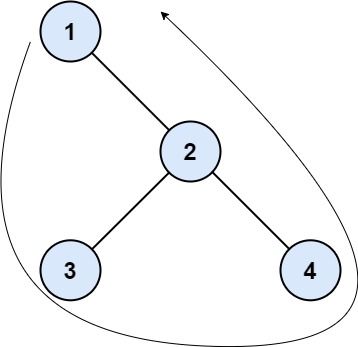
Example 1:
Input: root = [1,null,2,3,4] Output: [1,3,4,2] Explanation: - The left boundary is empty because the root does not have a left child. - The right boundary follows the path starting from the root's right child 2 -> 4. 4 is a leaf, so the right boundary is [2]. - The leaves from left to right are [3,4]. Concatenating everything results in [1] + [] + [3,4] + [2] = [1,3,4,2].
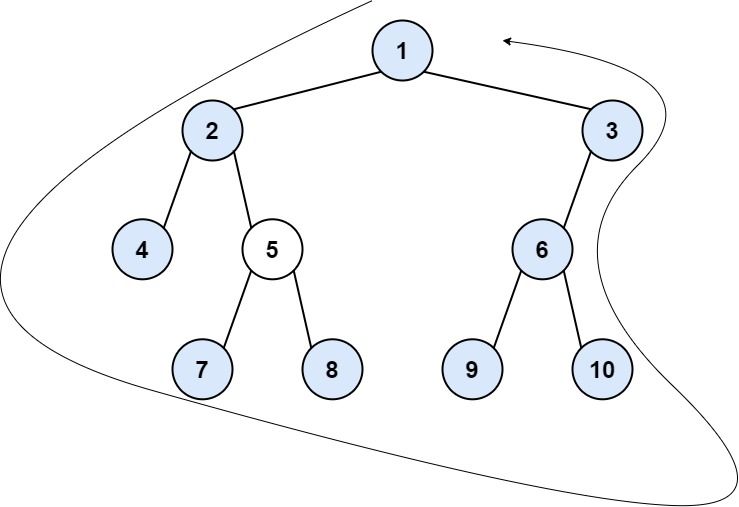
Example 2:
Input: root = [1,2,3,4,5,6,null,null,null,7,8,9,10] Output: [1,2,4,7,8,9,10,6,3] Explanation: - The left boundary follows the path starting from the root's left child 2 -> 4. 4 is a leaf, so the left boundary is [2]. - The right boundary follows the path starting from the root's right child 3 -> 6 -> 10. 10 is a leaf, so the right boundary is [3,6], and in reverse order is [6,3]. - The leaves from left to right are [4,7,8,9,10]. Concatenating everything results in [1] + [2] + [4,7,8,9,10] + [6,3] = [1,2,4,7,8,9,10,6,3].
Constraints:
- The number of nodes in the tree is in the range
[1, 104]. -1000 <= Node.val <= 1000
First, if the tree has only one node, we directly return a list with the value of that node.
Otherwise, we can use depth-first search (DFS) to find the left boundary, leaf nodes, and right boundary of the binary tree.
Specifically, we can use a recursive function
The function implementation is as follows:
- If
$\textit{root}$ is null, then directly return. - If
$\textit{i} = 0$ , we need to find the left boundary. If$\textit{root}$ is not a leaf node, we add the value of$\textit{root}$ to$\textit{nums}$ . If$\textit{root}$ has a left child, we recursively call the$\textit{dfs}$ function, passing in$\textit{nums}$ , the left child of$\textit{root}$ , and$\textit{i}$ . Otherwise, we recursively call the$\textit{dfs}$ function, passing in$\textit{nums}$ , the right child of$\textit{root}$ , and$\textit{i}$ . - If
$\textit{i} = 1$ , we need to find the leaf nodes. If$\textit{root}$ is a leaf node, we add the value of$\textit{root}$ to$\textit{nums}$ . Otherwise, we recursively call the$\textit{dfs}$ function, passing in$\textit{nums}$ , the left child of$\textit{root}$ and$\textit{i}$ , as well as$\textit{nums}$ , the right child of$\textit{root}$ and$\textit{i}$ . - If
$\textit{i} = 2$ , we need to find the right boundary. If$\textit{root}$ is not a leaf node, we add the value of$\textit{root}$ to$\textit{nums}$ . If$\textit{root}$ has a right child, we recursively call the$\textit{dfs}$ function, passing in$\textit{nums}$ , the right child of$\textit{root}$ , and$\textit{i}$ . Otherwise, we recursively call the$\textit{dfs}$ function, passing in$\textit{nums}$ , the left child of$\textit{root}$ , and$\textit{i}$ .
We call the
The time complexity is
# Definition for a binary tree node.
# class TreeNode:
# def __init__(self, val=0, left=None, right=None):
# self.val = val
# self.left = left
# self.right = right
class Solution:
def boundaryOfBinaryTree(self, root: Optional[TreeNode]) -> List[int]:
def dfs(nums: List[int], root: Optional[TreeNode], i: int):
if root is None:
return
if i == 0:
if root.left != root.right:
nums.append(root.val)
if root.left:
dfs(nums, root.left, i)
else:
dfs(nums, root.right, i)
elif i == 1:
if root.left == root.right:
nums.append(root.val)
else:
dfs(nums, root.left, i)
dfs(nums, root.right, i)
else:
if root.left != root.right:
nums.append(root.val)
if root.right:
dfs(nums, root.right, i)
else:
dfs(nums, root.left, i)
ans = [root.val]
if root.left == root.right:
return ans
left, leaves, right = [], [], []
dfs(left, root.left, 0)
dfs(leaves, root, 1)
dfs(right, root.right, 2)
ans += left + leaves + right[::-1]
return ansclass Solution {
public List<Integer> boundaryOfBinaryTree(TreeNode root) {
List<Integer> ans = new ArrayList<>();
ans.add(root.val);
if (root.left == root.right) {
return ans;
}
List<Integer> left = new ArrayList<>();
List<Integer> leaves = new ArrayList<>();
List<Integer> right = new ArrayList<>();
dfs(left, root.left, 0);
dfs(leaves, root, 1);
dfs(right, root.right, 2);
ans.addAll(left);
ans.addAll(leaves);
Collections.reverse(right);
ans.addAll(right);
return ans;
}
private void dfs(List<Integer> nums, TreeNode root, int i) {
if (root == null) {
return;
}
if (i == 0) {
if (root.left != root.right) {
nums.add(root.val);
if (root.left != null) {
dfs(nums, root.left, i);
} else {
dfs(nums, root.right, i);
}
}
} else if (i == 1) {
if (root.left == root.right) {
nums.add(root.val);
} else {
dfs(nums, root.left, i);
dfs(nums, root.right, i);
}
} else {
if (root.left != root.right) {
nums.add(root.val);
if (root.right != null) {
dfs(nums, root.right, i);
} else {
dfs(nums, root.left, i);
}
}
}
}
}/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode() : val(0), left(nullptr), right(nullptr) {}
* TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
* TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
* };
*/
class Solution {
public:
vector<int> boundaryOfBinaryTree(TreeNode* root) {
auto dfs = [&](this auto&& dfs, vector<int>& nums, TreeNode* root, int i) -> void {
if (!root) {
return;
}
if (i == 0) {
if (root->left != root->right) {
nums.push_back(root->val);
if (root->left) {
dfs(nums, root->left, i);
} else {
dfs(nums, root->right, i);
}
}
} else if (i == 1) {
if (root->left == root->right) {
nums.push_back(root->val);
} else {
dfs(nums, root->left, i);
dfs(nums, root->right, i);
}
} else {
if (root->left != root->right) {
nums.push_back(root->val);
if (root->right) {
dfs(nums, root->right, i);
} else {
dfs(nums, root->left, i);
}
}
}
};
vector<int> ans = {root->val};
if (root->left == root->right) {
return ans;
}
vector<int> left, right, leaves;
dfs(left, root->left, 0);
dfs(leaves, root, 1);
dfs(right, root->right, 2);
ans.insert(ans.end(), left.begin(), left.end());
ans.insert(ans.end(), leaves.begin(), leaves.end());
ans.insert(ans.end(), right.rbegin(), right.rend());
return ans;
}
};/**
* Definition for a binary tree node.
* type TreeNode struct {
* Val int
* Left *TreeNode
* Right *TreeNode
* }
*/
func boundaryOfBinaryTree(root *TreeNode) []int {
ans := []int{root.Val}
if root.Left == root.Right {
return ans
}
left, leaves, right := []int{}, []int{}, []int{}
var dfs func(nums *[]int, root *TreeNode, i int)
dfs = func(nums *[]int, root *TreeNode, i int) {
if root == nil {
return
}
if i == 0 {
if root.Left != root.Right {
*nums = append(*nums, root.Val)
if root.Left != nil {
dfs(nums, root.Left, i)
} else {
dfs(nums, root.Right, i)
}
}
} else if i == 1 {
if root.Left == root.Right {
*nums = append(*nums, root.Val)
} else {
dfs(nums, root.Left, i)
dfs(nums, root.Right, i)
}
} else {
if root.Left != root.Right {
*nums = append(*nums, root.Val)
if root.Right != nil {
dfs(nums, root.Right, i)
} else {
dfs(nums, root.Left, i)
}
}
}
}
dfs(&left, root.Left, 0)
dfs(&leaves, root, 1)
dfs(&right, root.Right, 2)
ans = append(ans, left...)
ans = append(ans, leaves...)
for i := len(right) - 1; i >= 0; i-- {
ans = append(ans, right[i])
}
return ans
}/**
* Definition for a binary tree node.
* class TreeNode {
* val: number
* left: TreeNode | null
* right: TreeNode | null
* constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) {
* this.val = (val===undefined ? 0 : val)
* this.left = (left===undefined ? null : left)
* this.right = (right===undefined ? null : right)
* }
* }
*/
function boundaryOfBinaryTree(root: TreeNode | null): number[] {
const ans: number[] = [root.val];
if (root.left === root.right) {
return ans;
}
const left: number[] = [];
const leaves: number[] = [];
const right: number[] = [];
const dfs = function (nums: number[], root: TreeNode | null, i: number) {
if (!root) {
return;
}
if (i === 0) {
if (root.left !== root.right) {
nums.push(root.val);
if (root.left) {
dfs(nums, root.left, i);
} else {
dfs(nums, root.right, i);
}
}
} else if (i === 1) {
if (root.left === root.right) {
nums.push(root.val);
} else {
dfs(nums, root.left, i);
dfs(nums, root.right, i);
}
} else {
if (root.left !== root.right) {
nums.push(root.val);
if (root.right) {
dfs(nums, root.right, i);
} else {
dfs(nums, root.left, i);
}
}
}
};
dfs(left, root.left, 0);
dfs(leaves, root, 1);
dfs(right, root.right, 2);
return ans.concat(left).concat(leaves).concat(right.reverse());
}/**
* Definition for a binary tree node.
* function TreeNode(val, left, right) {
* this.val = (val===undefined ? 0 : val)
* this.left = (left===undefined ? null : left)
* this.right = (right===undefined ? null : right)
* }
*/
/**
* @param {TreeNode} root
* @return {number[]}
*/
var boundaryOfBinaryTree = function (root) {
const ans = [root.val];
if (root.left === root.right) {
return ans;
}
const left = [];
const leaves = [];
const right = [];
const dfs = function (nums, root, i) {
if (!root) {
return;
}
if (i === 0) {
if (root.left !== root.right) {
nums.push(root.val);
if (root.left) {
dfs(nums, root.left, i);
} else {
dfs(nums, root.right, i);
}
}
} else if (i === 1) {
if (root.left === root.right) {
nums.push(root.val);
} else {
dfs(nums, root.left, i);
dfs(nums, root.right, i);
}
} else {
if (root.left !== root.right) {
nums.push(root.val);
if (root.right) {
dfs(nums, root.right, i);
} else {
dfs(nums, root.left, i);
}
}
}
};
dfs(left, root.left, 0);
dfs(leaves, root, 1);
dfs(right, root.right, 2);
return ans.concat(left).concat(leaves).concat(right.reverse());
};