Skip to content

Latest commit

 

History

History
204 lines (172 loc) · 6.67 KB

README_EN.md

File metadata and controls

204 lines (172 loc) · 6.67 KB
comments difficulty edit_url rating source tags
true
Hard
2062
Weekly Contest 271 Q4
Array
Binary Search
Prefix Sum
Sliding Window

2106. Maximum Fruits Harvested After at Most K Steps

中文文档

Description

Fruits are available at some positions on an infinite x-axis. You are given a 2D integer array fruits where fruits[i] = [positioni, amounti] depicts amounti fruits at the position positioni. fruits is already sorted by positioni in ascending order, and each positioni is unique.

You are also given an integer startPos and an integer k. Initially, you are at the position startPos. From any position, you can either walk to the left or right. It takes one step to move one unit on the x-axis, and you can walk at most k steps in total. For every position you reach, you harvest all the fruits at that position, and the fruits will disappear from that position.

Return the maximum total number of fruits you can harvest.

 

Example 1:

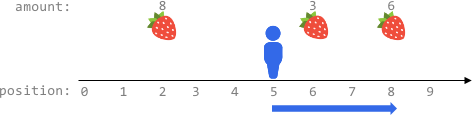
Input: fruits = [[2,8],[6,3],[8,6]], startPos = 5, k = 4
Output: 9
Explanation: 
The optimal way is to:
- Move right to position 6 and harvest 3 fruits
- Move right to position 8 and harvest 6 fruits
You moved 3 steps and harvested 3 + 6 = 9 fruits in total.

Example 2:

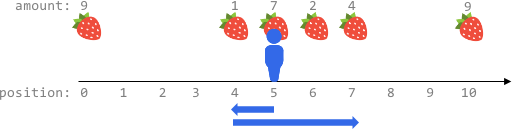
Input: fruits = [[0,9],[4,1],[5,7],[6,2],[7,4],[10,9]], startPos = 5, k = 4
Output: 14
Explanation: 
You can move at most k = 4 steps, so you cannot reach position 0 nor 10.
The optimal way is to:
- Harvest the 7 fruits at the starting position 5
- Move left to position 4 and harvest 1 fruit
- Move right to position 6 and harvest 2 fruits
- Move right to position 7 and harvest 4 fruits
You moved 1 + 3 = 4 steps and harvested 7 + 1 + 2 + 4 = 14 fruits in total.

Example 3:

Input: fruits = [[0,3],[6,4],[8,5]], startPos = 3, k = 2
Output: 0
Explanation:
You can move at most k = 2 steps and cannot reach any position with fruits.

 

Constraints:

  • 1 <= fruits.length <= 105
  • fruits[i].length == 2
  • 0 <= startPos, positioni <= 2 * 105
  • positioni-1 < positioni for any i > 0 (0-indexed)
  • 1 <= amounti <= 104
  • 0 <= k <= 2 * 105

Solutions

Solution 1

Python3

class Solution:
    def maxTotalFruits(self, fruits: List[List[int]], startPos: int, k: int) -> int:
        ans = i = s = 0
        for j, (pj, fj) in enumerate(fruits):
            s += fj
            while (
                i <= j
                and pj
                - fruits[i][0]
                + min(abs(startPos - fruits[i][0]), abs(startPos - fruits[j][0]))
                > k
            ):
                s -= fruits[i][1]
                i += 1
            ans = max(ans, s)
        return ans

Java

class Solution {
    public int maxTotalFruits(int[][] fruits, int startPos, int k) {
        int ans = 0, s = 0;
        for (int i = 0, j = 0; j < fruits.length; ++j) {
            int pj = fruits[j][0], fj = fruits[j][1];
            s += fj;
            while (i <= j
                && pj - fruits[i][0]
                        + Math.min(Math.abs(startPos - fruits[i][0]), Math.abs(startPos - pj))
                    > k) {
                s -= fruits[i++][1];
            }
            ans = Math.max(ans, s);
        }
        return ans;
    }
}

C++

class Solution {
public:
    int maxTotalFruits(vector<vector<int>>& fruits, int startPos, int k) {
        int ans = 0, s = 0;
        for (int i = 0, j = 0; j < fruits.size(); ++j) {
            int pj = fruits[j][0], fj = fruits[j][1];
            s += fj;
            while (i <= j && pj - fruits[i][0] + min(abs(startPos - fruits[i][0]), abs(startPos - pj)) > k) {
                s -= fruits[i++][1];
            }
            ans = max(ans, s);
        }
        return ans;
    }
};

Go

func maxTotalFruits(fruits [][]int, startPos int, k int) (ans int) {
	var s, i int
	for j, f := range fruits {
		s += f[1]
		for i <= j && f[0]-fruits[i][0]+min(abs(startPos-fruits[i][0]), abs(startPos-f[0])) > k {
			s -= fruits[i][1]
			i += 1
		}
		ans = max(ans, s)
	}
	return
}

func abs(x int) int {
	if x < 0 {
		return -x
	}
	return x
}

TypeScript

function maxTotalFruits(fruits: number[][], startPos: number, k: number): number {
    let ans = 0;
    let s = 0;
    for (let i = 0, j = 0; j < fruits.length; ++j) {
        const [pj, fj] = fruits[j];
        s += fj;
        while (
            i <= j &&
            pj -
                fruits[i][0] +
                Math.min(Math.abs(startPos - fruits[i][0]), Math.abs(startPos - pj)) >
                k
        ) {
            s -= fruits[i++][1];
        }
        ans = Math.max(ans, s);
    }
    return ans;
}